932. The height of triangle
The area of triangle is S.
The length of its base is a greater
than its height. Find the height of triangle.
Input. Two integers: S (0 < S ≤ 100) and a (|a| ≤ 100).
Output. Print the height of triangle
with two digits after the decimal point.
|
Sample input |
Sample output |
|
15 1 |
5.00 |
SOLUTION
geometry - elementary
Algorithm analysis
Let h be the
height of triangle. Then its base is h + a. The area of the triangle is S = ½ h (h
+ a). The values of S and a are
given, solve the quadratic equation for h:
h2 + ha – 2S = 0,
discriminant D = a2 + 4S,
the positive root is h = 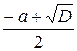
Algorithm realization
Read the input data. Solve the quadratic equation S = ½ h (h
+ a) for h and take its positive root.
scanf("%lf %lf",&s,&a);
d
= a*a + 8*s;
h
= (-a + sqrt(d)) / 2;
printf("%.2lf\n",h);
Java realization
import java.util.*;
public class Main
{
public static void main(String[] args)
{
Scanner con = new Scanner(System.in);
double s = con.nextDouble();
double a = con.nextDouble();
double d = a * a + 8 * s;
double h = (-a + Math.sqrt(d)) / 2;
System.out.println(h);
con.close();
}
}
Python realization
import math
s, a = map(float,input().split())
d = a*a + 8*s;
h = (-a + math.sqrt(d)) / 2;
print("%.2f"
%h)