1782. The sum of divisors
Let n be a positive integer. The number d is its divisor if 1 ≤ d ≤ n and the remainder after dividing n by d is zero. Find the
sum of divisors of number n.
Input. One integer n (1 ≤ n
≤ 1018), all its prime divisors do not exceed 1000.
Output. Print the
sum of divisors of number n.
|
Sample
input |
Sample
output |
|
239 |
240 |
SOLUTION
number theory
Algorithm analysis
Let σ(n) be the function that computes the sum of all
divisors of
number n.
·
If n = p (p is prime), then σ(n) = 1 + p;
·
If n = pa (p is prime), then σ(pa) = 1 + p + p2 + p3 + … + pa = 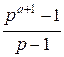 ;
;
Function σ(n) is multiplicative. If n = ![]() , then
, then
σ(n) = σ(![]() ) * σ(
) * σ(![]() ) * … * σ(
) * … * σ(![]() ) =
) = 
This sum can also be written in the form
σ(n) = 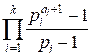 =
= 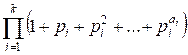 =
= 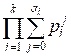
Example
n = 239 is prime, σ(239) = 1 + 239 = 240.
n = 24 = 23 * 3, σ(24) = σ(23) * σ(3) = (1 + 2 + 4 + 8) * (1 +
3) = 15 * 4 = 60.
Let’s calculate the sum of
divisors for n = 24 directly: σ(24) =
1 + 2 + 3 + 4 + 6 + 8 + 12 + 24 =
1 + 2 + 4 + 8 +
3 * (1 + 2 + 4 + 8) =
(1 + 2 + 4 + 8)
* (1 + 3) = 15
* 4 = 60
Algorithm realization
Read the input value of n.
scanf("%lld",&n);
res = 1;
In the variable i iterate over
all prime divisors of n (according to
the problem statement, they are no more than 1000). Calculate the sum of divisors of n using the formula given in the analysis
of the problem.
for(i = 2; i <= 1000; i++)
{
p = i;
Let i be a prime
divisor of n. Find
the maximum power of a for which ia
divides n.
while(n % i
== 0)
{
n /= i;
p *= i;
}
After the while
loop we have p = ia+1.
Multiply res by ![]() .
.
res *= (p - 1) / (i - 1);
}
Print the answer.
printf("%lld\n",res);
Java realization
import java.util.*;
class Main
{
public static void main(String[] args)
{
Scanner con = new Scanner(System.in);
long n = con.nextLong();
long res = 1;
for(int i = 2; i <= 1000; i++)
{
long p = i;
while(n % i == 0)
{
n /= i;
p *= i;
}
res *= (p - 1) / (i - 1);
}
System.out.println(res);
con.close();
}
}
Python realization
n = int(input())
res = 1
for i in range (2,1000) :
p = i
while(n % i
== 0) :
n = n // i
p *= i
res *= (p - 1) // (i - 1)
print(res)