7714. Milling machines
A Fab Lab is a
small open workshop where you can create or manufacture almost anything you
want, mainly using computer-controlled tools such as a laser cutter or a 3D
printer. Recently, the FAU Fab Lab acquired a CNC milling machine. With this
machine, you can cut or remove material from the surface of a workpiece using
various tools. The entire process is controlled by a computer program.
Sometimes I
wondered what would happen if several workpieces of different shapes were
processed using the same milling program. To simplify the situation, let’s
assume that we only have two-dimensional workpieces without holes. A milling
program consists of several steps, each describing which parts of the surface
the milling machine should remove material from (using different tools).
Input. The first line contains two integers w and s (1 ≤ w, s ≤ 104)
– the number of workpieces and the number of steps in the milling program.
The next line contains two
integers x and y (1 ≤ x, y ≤ 100),
where x is the width of a workpiece,
and y is its maximum possible height.
Each of the
following w lines describes one
workpiece. The description of each workpiece consists of x non-negative integers defining the
surface height in each column.
Then follow s lines describing the milling
steps. Each milling step consists of x non-negative
integers si (0 ≤ si ≤ y), determining
how much material should be removed in each column (relative to the milling
area height, i.e., relative to y,
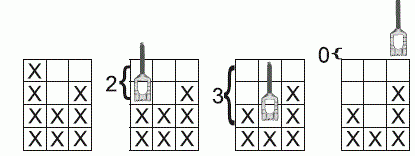
not to the top of the workpiece). See the illustration.
Output. For each workpiece, print one line
containing integers – the remaining surface heights after all
milling steps (in the same order as in the input).
Figure. In the first example, you can see how the
second workpiece looks after applying all milling steps sequentially: each
value in the program defines the vertical position of the milling head.
|
Sample
input 1 |
Sample
output 1 |
|
2 1 3 4 4 4 4 4 2 3 2 3 0 |
2 1 4 2 1 3 |
|
|
|
|
Sample
input 2 |
Sample
output 2 |
|
1 3 10 100 11 22 33 44 55 66 77 88 99 100 1 100 1 100 1 100 1 100 1 100 58 58 58 58 58 58 58 58 58 58 42 42 42 42
42 42 42 42 66 42 |
11 0 33 0 42 0 42 0 34 0 |
SOLUTION
mathematics
Algorithm analysis
The milling
program consists of s steps. Let at
the ![]() -th step (
-th step (![]() ) a layer of
thickness mij be removed
from the surface in column j (1
≤ j ≤ x). It is clear that the total amount
removed in column j will be
) a layer of
thickness mij be removed
from the surface in column j (1
≤ j ≤ x). It is clear that the total amount
removed in column j will be
max(m1j, m2j, …, msj)
We’ll call the
milling scheme the set of integers
(cuts1, cuts2, …, cutsx),
where
cutsj = max(m1j, m2j, …, msj), 1 ≤ j ≤ x

The same milling
scheme is applied to all w workpieces. After computing the values of cutsj (1 ≤ j ≤ x), this scheme is
then applied sequentially to each workpiece.
Algorithm implementation
Declare the arrays. The information about the workpieces is stored in the
array detail, and the milling scheme is stored in the array cuts.
int detail[10001][101], cuts[101];
Read the input data.
scanf("%d %d %d %d",
&w, &s, &x, &y);
Read the information about the w workpieces.
for (i = 0; i < w; i++)
for (j = 0; j < x; j++)
scanf("%d",
&detail[i][j]);
Read and process the s steps of
the milling program. For each position j,
the value cuts[j] represents the
maximum cutting depth achieved at any of the steps.
for (i = 0; i < s; i++)
for (j = 0; j < x; j++)
{
scanf("%d", &val);
if (val > cuts[j]) cuts[j] = val;
}
The maximum possible height of a workpiece is y. The milling head at position j is lowered to a
depth of cuts[j]. Consequently,
after milling, the remaining height of the i-th workpiece at position j will be equal to
min(detail[i][j],
y – cuts[j]).
for (i = 0; i < w; i++)
{
for (j = 0; j < x; j++)
printf("%d ", min(detail[i][j], y - cuts[j]));
printf("\n");
}